A fibration model for $d$-dimensional image patches
Brad Nelson
Department of Statistics & CCAM
University of Chicago
AMS Special Session on Applied Topology
January 8, 2021
bnels.github.io/jmm2021
Acknowledgement/Support
Thanks to Gunnar Carlsson for many discussions on the Klein bottle for image patches and possible extensions.
Funding I received while working on this topic
- DoD through NDSEG fellowship
- DOE through SLAC/Neutrino group
- Unbox AI
Outline
- Image Patch Data
- Data Exploration
- Fibration Model
- Fibration-Assisted Visualization
Image Patch Data
2D: $\ell \times \ell$ pixel squares.
3D: $\ell \times \ell \times \ell$ voxel cubes.
Patches are sampled from natural (non-random) images, so there is structure in the data.
Filter for high-contrast, dense subsets.
k, p: take top p% ranked by distance to k-nearest neighbor.
Topology of Image Patches
- [LPM 02] find annulus when investigating patch statistics
- Preliminary topological investigations in [dSC 04]
- Klein bottle model proposed in [C+ 08]
- Compression [MSC 08] Texture recognition [PC 14] Neural nets [GC 19]
- Range images [AC 09] Flow [A+ 20]
Topology in the visual cortex
- [T 95] Klein bottle model in stimulus maps.
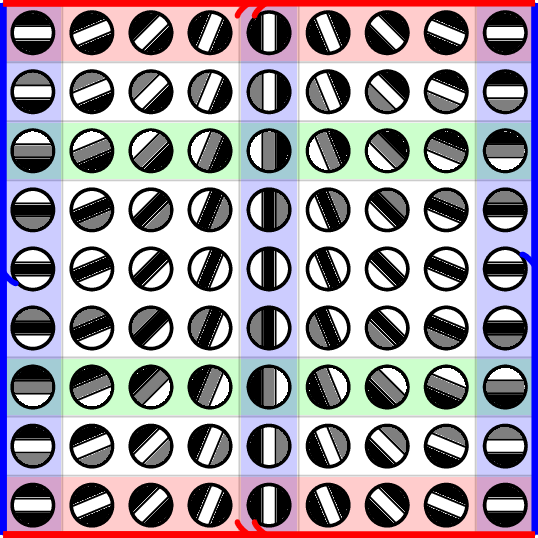
Klein Bottle In Image Patches

Contributions
- Topological modeling of 3D image patch data.
- Analyze a fiber bundle model that generalizes Klein bottle for 2D patches.
- Use this to understand the distribution of patches in different data sets.
Data
Van Hateren data set [vHvdS 98] - data base of 2D natural images.
BRATS MRI data set [BRATS]
Penobscot seismic interpetation data [Pen]

Data Processing
Procedure used in [LPM 02]
- Sample random patches
- Take top 20% by contrast norm
- Mean-center and normalize contrast
Additional steps used for high-density regions [dSC 04, C+ 08]
- Compute distances to $k$-nearest neighbor
- Take $p$% by smallest distance
Eigenpatches = principal components interpreted as patch.
Van Hateren, k=100 p=20
2-dimensional PCA embedding with eigenpatches.


$7\times 7$ patches
Primary circle [dSC 04]
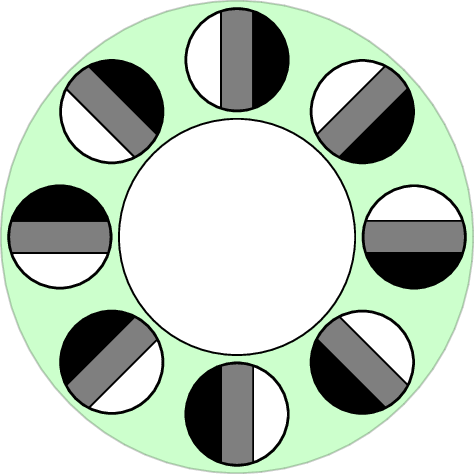
Penobscot, k=30 p=40
2-dimensional PCA embedding with eigenpatches.

$5\times 5\times 5$ patches
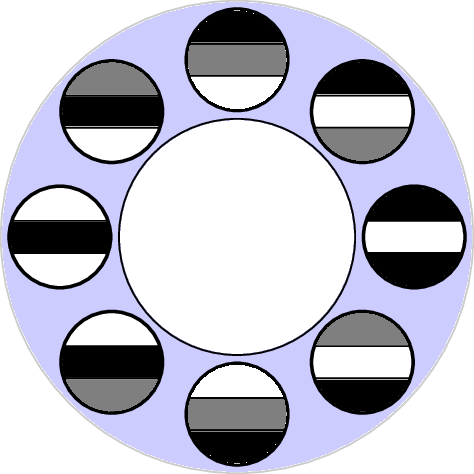
BRATS k=100 p=40
3-dimensional PCA embedding with eigenpatches.PCA 1
$5\times 5\times 5$ patches
PCA 2
The model space $\cal K^d$
Consider $d$-dimensional patches.
We'll consider idealized patches as functions on the disk $D^d$
We'd like to capture primary spheres and secondary circles in a topological model.

The primary $(d-1)$ sphere [dSC 04]:
$\{(v_\phi^T x) \mid v_\phi\in S^{d-1}\}$
The model space $\cal K^d$
Fix $v_\phi \in S^{d-1}$. A secondary circle [dSC 04]:
$\{\cos(\theta)(v_\phi^T x)^2 + \sin(\theta)(v_\phi^T x)\mid \theta \in [0,2\pi)\}$

Note that $v_\phi$ and $-v_\phi$ produce the same secondary circle.
The model space $\cal K^d$
$\mathcal{K}^d = \{ k(v_\phi, \theta; x) = \cos(\theta)(v_\phi^T x)^2 + \sin(\theta)(v_\phi^T x) \mid v_\phi\in S^{d-1}, \theta \in [0,2\pi) \}$
There is an identification $k(-v_\phi, -\theta;x) = k(v_\phi, \theta; x)$

$\mathcal{K}^2$ is a Klein bottle [C+ 08]. $\mathcal{K}^3$ is a $3$-dimensional manifold.
The Harris Map
Harris corner/edge detector [HS 88] analyzes eigenvalues of:
$M(x) = \sum_i \Delta(x_i) \Delta(x_i)^T$, $\Delta$ finite difference gradient.
Harris map $h: x\mapsto \text{MaxEigVec}(M(x))$
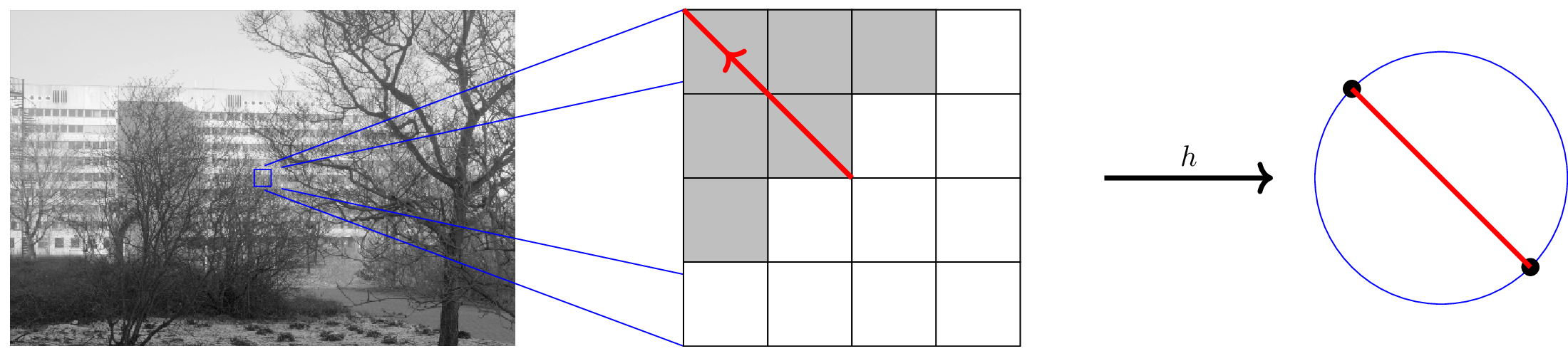
There is a sign/scale ambiguity for eigenvectors, so range is naturally $\mathbb{R}P^{d-1}$
We also see this ambiguity in our "secondary circles".
The Harris Map
Continuous limit
$M(f(x)) = \int_x dx\, \nabla(f(x))\nabla(f(x))^T$
For $k(v_\phi, \theta; x)\in \mathcal{K}^d$, $\nabla(k(x)) = k'(x) v_\phi$
so $M(k(x))$ is rank-1, and $h(k(v_\phi, \theta; x)) = [v_\phi]$
$\mathcal{K}^d$ is a fiber bundle over $\mathbb{R}P^{d-1}$
(non-trivial because of twist in identification)
The Harris map $h$ is a fibration for $\mathcal{K}^d$
Fibers $h^{-1}([v])$ are secondary circles.
Homology of $\cal K^d$
Because of fibration structure, we can use Leray-Serre spectral sequence to compute homology.
We'll use integer ($\mathbb{Z}$) coefficients, which can be used to obtain field coefficients.
$H_\ast(\mathcal{K}^2) = (\mathbb{Z}, \mathbb{Z}\oplus \mathbb{Z}_2, 0)$
$H_\ast(\mathcal{K}^3) = (\mathbb{Z}, \mathbb{Z}_2\oplus \mathbb{Z}_2, 0, \mathbb{Z})$
See [N 20] Theorem 5.2.6 for detailed calculations.
Fibration-Assisted Visualization
3D patches are high dimensional (125 dimensions for 5x5x5).
PCA can visualize some simple structures.
$\mathcal{K}^3$ is a 3-dimensional manifold that captures more.
Visualize data using affine space of $\mathcal{K}^3$
- $xy$-plane: Stereographic projection of $\mathbb{R}P^2$ base space
- $z$-axis: $S^1$ fiber cut at $2\pi$
BRATS patches on $\cal K^3$
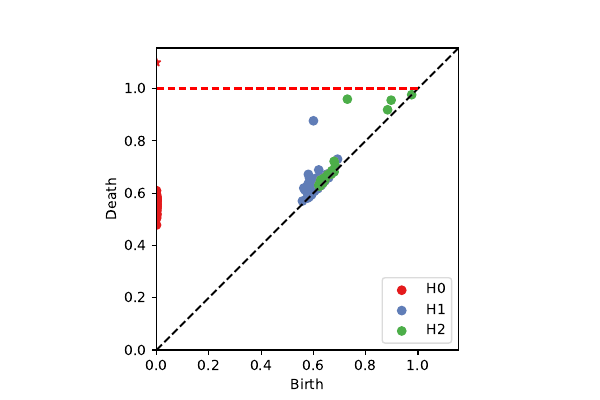 k=100, p=40: 2-sphere + circle (chord)
k=100, p=40: 2-sphere + circle (chord)
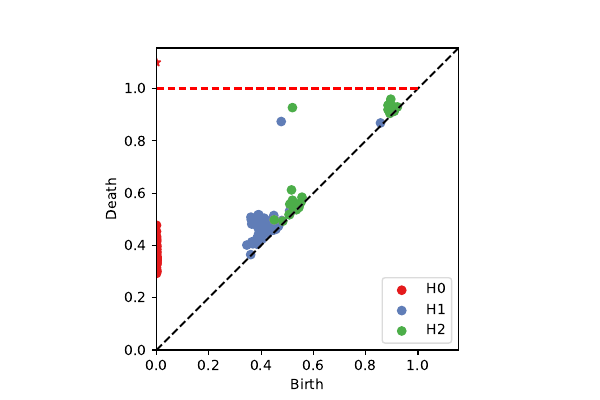 projection onto $\mathcal{K}^3$
projection onto $\mathcal{K}^3$
Penobscot patches on $\cal K^3$
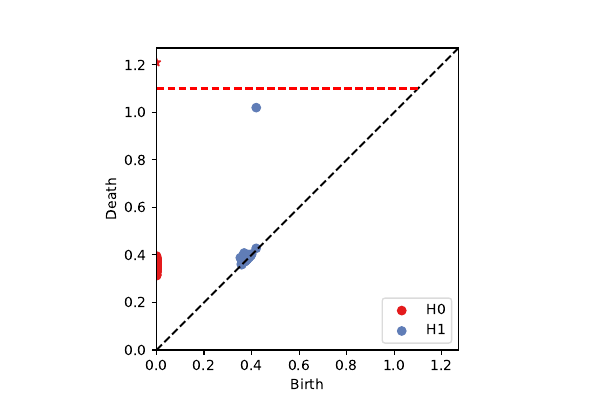 k=100, p=40: secondary circle
k=100, p=40: secondary circle
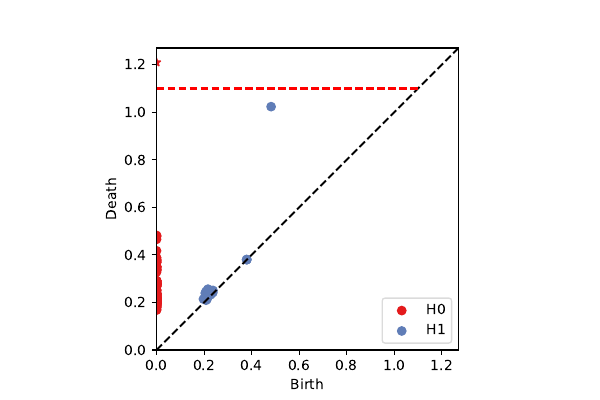 projection onto $\mathcal{K}^3$
projection onto $\mathcal{K}^3$
Review & Future Directions
We've developed a model $\cal K^d$ to explain structures we see in image patch data.
- Useful for interpreting subsets of the data.
- Captures topology of high-dimensional data with small number of dimensions.
For additional details, see [N 20] "Parameterized Topological Data Analysis."
Potential applications: 3D compression, texture, neural nets [MSC 08, PC 14, GC 19]
If we have higher-dimensional patches, we could perform similar analyses.
Where would a 4-dimensional image come from?
Other applications where we might use a map:
Evasion [AC 14],
Time series,
Control systems.
Technical Credits
Bibliography
[N 20] B. Nelson. Parameterized Topological Data Analysis.
PhD Dissertation, Stanford University. 2020. (Link)
[AC 09] Adams, Carlsson. On the Nonlinear Statistics of Range Image Patches. 2009.
[AC 14] - Adams, Carlsson. Evasion Paths in Mobile Sensor Networks. 2015.
[BRATS] BRATS data set. https://www.med.upenn.edu/sbia/brats2018/registration.html
[C+ 08] Carlsson, Ishkhanov, de Silva, Zomorodian. On the Local Behavior of Spaces of Natural Images. 2008.
[dSC 04] de Silva, Carlsson. Topological estimation using witness complexes. 2004.
[GC 19] Gabrielsson, Carlsson. Exposition and Interpretation of the Topology of Neural Networks. 2019.
[LPM 02] Lee, Pedersen, Mumford. The Nonlinear Statistics of High-Contrast Patches in Natural Images. 2002.
[MSC 08] Maleki, Shahram, Carlsson. A Near Optimal Coder For Image Geometry With Adaptive Partitioning. 2008.
[PC 14] Perea, Carlsson. A Klein-Bottle-Based Dictionary for Texture Representation. 2014.
[Pen] Penobscot Interpretation Dataset. https://zenodo.org/record/1341774
[T 95] Tanaka. Topological Analysis of Point Singularities in Stimulus Preference Maps of the Primary Visual Cortex. 1995.
[vHvdS 98] van Hateren, van der Schaaf. Independent component filters of natural images compared with simple cells in primary visual cortex. 1998.